Measuring source signatures
Introduction
Signature measurement
Marine seismic source signatures have historically been measured in very simple ways, usually involving two parameters, both of which are strongly dependent on the bandwidth in which the signature is measured so any values quoted without bandwidth are essentially meaningless. The two parameters are:-
Amplitude
This is a simple measurement of the strength of the source. It can be measured close up or far away as follows.
-
Far-field signature. This is by the most common form. It is a measure of the signature a long way from the source and vertically underneath but with the amplitude referred back to 1m from the source. In other words, the units used to measure this are bar-m. The far-field signature always includes the effects of the ghost reflection.
-
Near-field signature. This is a measure of the amplitude of the source at some fixed position close by the source, usually 1m. away from it. For multiple guns it is usually measured from the geometric centre a little underneath the source. The natural units for this are bars. If the measurement is close to the source, the ghost reflection is usually much smaller than the direct arrival because of geometric spreading. (The direct arrival might travel 1m but the ghost travels maybe 12m if the gun or array is deployed at 6m depth). A single near-field recording of a whole array is difficult to relate to anything useful so far-field signatures are by far the most common for arrays. Near or far-field signatures might be given for a single gun or a cluster.)
Amplitude is often used as a measure of penetration of an array but this is a rather unsophisticated viewpoint. Different frequencies travel very different distances in the earth due to Q-filtering so penetration is a much more complex question. It is quite possible that seismic arrays are sometimes too strong for their stated goals. Deep penetration in the earth, particularly in places where considerable absorption takes place (low Q) such as basalt, requires an abundance of low frequencies, normally achieved with airgun arrays by deploying them more deeply.
Primary to bubble ratio
Primary to bubble ratio is related to deconvolvability. The bubble is an artifact in that the ideal source is a spike. Much effort has been expended over the years into reducing the bubble relative to the peak to approach the idealised spike more closely. However, this again has probably attracted more attention than it need as Wiener deconvolution is often highly capable at compressing the signature provided the primary to bubble is relatively modest, (anything above about 5 in the seismic band works very well).
It should be noted that measuring the primary to bubble ratio is sometimes not so simple. A fundamental requirement for measuring this parameter is to identify where the bubble starts. Gundalf will start searching from 40 msec after the main peak although this default can be changed. Sometimes, this defeats any automated algorithm, particularly if signatures are narrow bandwidth and it may be necessary to judge the start time by eye.
The best way to do this is to start by trying to identify the bubble start in the UNfiltered signature. Filtering interferes with bubble identification in all kinds of subtle ways. With airgun arrays, the bubble is usually anywhere between about 80-140 msec after the initial peak. For most reasonably designed airgun arrays, it is easy to identify in the unfiltered case and will consist of a small peak followed by a small trough. This mirrors the main signature which starts with a large peak followed by the large ghost trough. If you can't identify it, this is simply telling you that it is not well-defined.
Assuming you can identify it, measure the time delay from the main peak to the bubble peak and back off a little. Then specify this as the time when Gundalf should start looking for the bubble.
The important thing is to use the same time delay for the filtered versions of the signature. This has a much better chance of producing a stable bubble identification and therefore a stable primary to bubble ratio.
Without this process, it is easy to get very variable estimates of primary to bubble. This is not a deficiency in Gundalf. It merely reflects the fact that primary to bubble can be an elusive parameter in modern airgun arrays with digital filtering because the bubble is normally suppressed so well.
It should finally be noted that primary to bubble itself can be calculated in two ways even when the bubble start has been correctly identified.
-
Peak to peak. If the peak and ghost trough are both well-developed with the ghost near the ideal value of -1, using the peak to peak values for both the primary and the bubble gives a better estimate.
-
Zero to peak. For a shallow source, the ghost may not be well developed due to anelastic behaviour at the surface. In this case, it may be more appropriate to use the zero to peak values for both primary and bubble to give a more robust estimate. This is also true with near-field measurements.
Amplitude spectral measurement
Amplitude spectral measurements are also quoted but not often. These may include some measure of the size of the ripples in some restricted bandwidth, say 15-70Hz. They may also include the bandwidth within 3 or 6db down from the spectral maximum.
Measurement examples
| Illustrating signature statistics, dfsv_6-18_180-72 Hz filtering. |
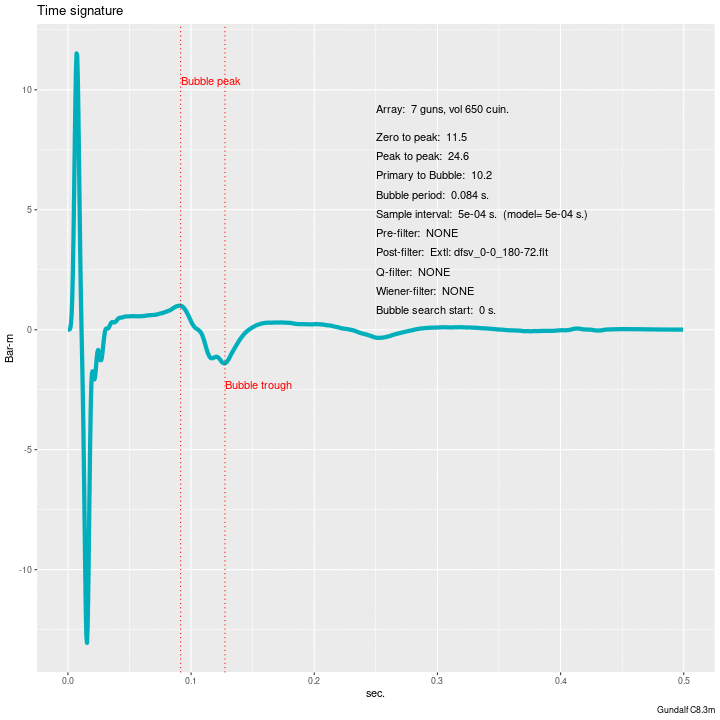
|
| Illustrating amplitude statistics, dfsv_6-18_180-72 Hz filtering. |
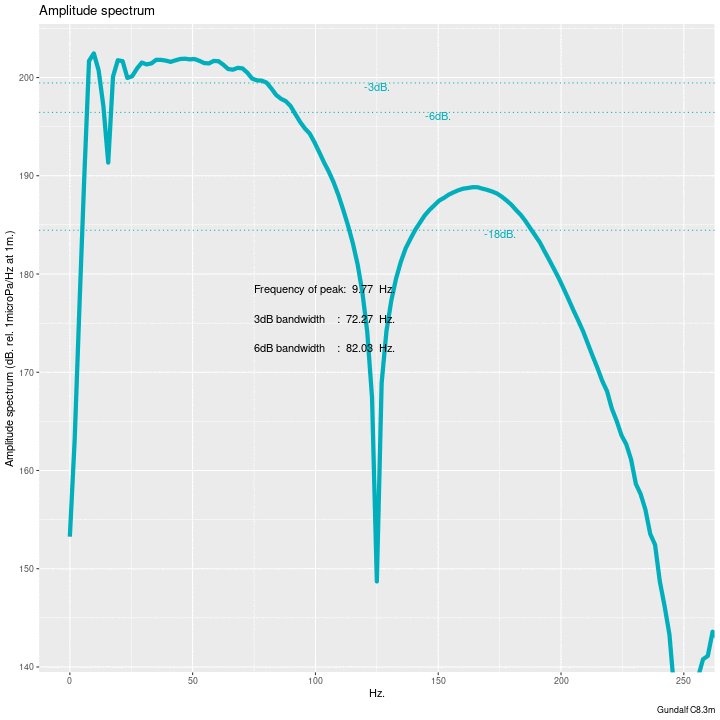
|